Superstucture on Lipid Membranes

Lipid membranes are the basic structure of all membranes of living cells. For an introduction into lipid membranes see my page about surfactants (or for the German version about "Amphiphile").

The physics of pure lipid bilayers without any proteins or other additions is complicated enough to start with. Such membranes are fluids in there lateral direction. Hence there is no shear rigidity. On the other hand the hydrophobic effect (i.e. the hydrophobicity of the tails) is so strong that there the stretching modulus is quite high. Hence stretching is relatively unimportant for lipid membranes. The remaining kind of elasticity is the bending elasticity. This is the most important one for lipid bilayers. It can be described by the famous Helfrich Hamiltonian[1]:

with c1,c2 being the fundamental curvatures, H the mean and K the Gaussian curvature. C0 is the spontaneous curvature. The two "kappas" are the bending rigidity and the Gaussian bending rigidity, respectively.

For most purpose the Gaussian part is constant since it depends only on the topology. Normally this Hamiltonian is sufficient. However in some cases high order term in the curvatures may become important. In 1989 Helfrich postulates a microscopic superstructure on lipid bilayers[2]. Unfortunately with the standard Hamiltonian every membrane structure apart form the plane costs energy. But since this structure should be really small (in comparison to the "normal" membrane structure one can see under the light microscope) one may need more term for a complete description[3].

In the same paper[2] Helfrich found out that the modulus of the quadratic gaussian curvature is negative. This means that on some scale saddlestructure will became favorable about the planar state of the membrane. My task was now to find this state, using simulated annealing based on the Monte-Carlo-Simulation of the membrane. In contrast to my PhD-thesis in this case I modeled by a surface parameterized in the Monge-representation. In order to gain numerical stability one has to add an additional term to prevent infinitely steep saddles. The term term I choose was the Gaussian curvature to the fourth. For the real one should keep in mind that there are 9 terms of fourth order in the curvature. Since I take only one of those into account for my model I need some to represent the sum of all this terms. One assumes that in the real systems the other 8 terms of 8 order will have the same stabilizing effect as the single eighth order term I used. With that said the the in my simulation the Hamiltonian looks like:

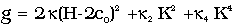
As a result I found a periodic saddle structure with broad saddles, minima and maxima, but steep edges in between [4]. I was able to deduce a the period of this structure to be between 4 an 20 nm depending on the value of the bending rigidity. For more details, see [4] or [5]. I expect newer paper by A. Jud and W. Helfrich which move on with the project after I left the Helfrich group to join the MPI.

Notes and Literature

1. W. Helfrich; Zeitschrift für Naturforschung c, 28, Heft 11/12: 693-703, 1973
2. W. Helfrich; Liquid Crystals, 1989, Vol. 5, No. 6, 1647-1658
3. If one compares this to the results of my PhD-thesis, namely with the analysis of the bending-elasticity, one may conclude that there are no additional term down to molecular scales. However this results were obtained through an analysis of the fluctuation modes. Nevertheless an underlying structure which flows around may average out. Hence this only thing to conclude from both of my results is that additional term must be small enough that they don't show up in the fluctuation spectrum even so the may modify the membranes shape groundstate.
4. R. Goetz and W.Helfrich; J. Phys. II France 6:215-23, 1996.
5. R. Goetz; "Monte-Carlo-Simulation einer Überstruktur auf Lipidmembranen", Diploma thesis (German only), 1993